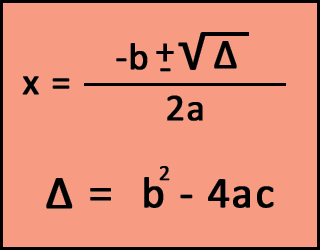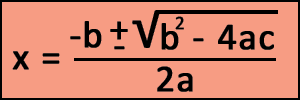The quadratic formula calculator is designed to determine the second-order polynomial quadratic equations.
The quadratic formula calculator is a free tool where you can easily solve the quadratic equations. Just enter the three values and get the discriminant, quadratic formula, first root, and second root in an instant.
The Quadratic name comes from "Quad" and we can also call it "Square" (Like: x2). It's a solution of a second-order polynomial equation with three coefficients.
We can write the equation in standard form like this:
Ax2 + Bx + C = 0 ( Where A ≠ 0 )
Here, A, B, and C are the Coefficients or Known Numbers.
Whereas, the 'x' is the unknown variable that we need to find using the quadratic formula.
The following are some examples for better understanding.
| 3x2 + 4x + 1 = 0 | Here a=3, b=4, and c=1 |
| 2x2 - 4x = 0 | You can see there is just 2 coefficients values at left side. So, we can figure our the values like this:
|
| 4x + 2 = 0 | It is not a quadratic equation. Because there is no x2 value. So, 'a' is counted as 0. |
Sometimes the quadratic equations are not in standard form. So, we need to move or expand some coefficients to convert it into standard form.
Let's see some examples:
| Disguise Form | Rearrangements | Standard Form | Coefficients |
|---|---|---|---|
| 2x2 = 5x - 4 | Move right values to left side | 2x2 - 5x + 4 = 0 | a=2, b=-5, c=4 |
| 4(y2 + 3y) = 2 | Expand left side and move 2 to left | 4y2 + 12y - 2 = 0 | a=4, b=12, c=-2 |
The quadratic formula is as below and it's used to find the "x" value.
Where,
Δ = b2 - 4ac
Our quadratic formula calculator determines the Discriminant values, whether it is greater than, less than, or equal to 0.
If b2 - 4ac is,
Let's take some examples to understand the process step by step.
Firstly, convert the equation into a standard form like this: ax2 + bx + c = 0.
Take -3 from right to left-hand side. As a result, we get:
So, we have:
Now place the known values in the quadratic formula.
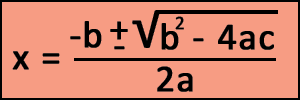
| x = |
|
| x = |
|
| x = |
|
As you can see, the discriminant (b2 - 4ac) = 1 that is > 0. So, there are two real roots.
For the First Root:
| x = |
|
x = -1
For the Second Root:
| x = |
|
x = -1.5
So, the roots of 2x2 + 5x + 3 = 0 are -1 and -1.5.
Here Coefficients are:
Firstly, let's find the discriminant (Δ):
Δ = b2 − 4ac
= 152 - 4 × (4 × 20)
= 152 - 4 × (80)
= 225 - 320 = -95
Here, the discriminant is -95. That is negative. So, we will get 2 complex roots.
Now let's find the roots.
| x = |
|
| x = |
|
√(-95) = 1.21835i ( Where i = √−1, Imaginary number )
| So, x = |
|
Finally, the First Root = -1.875 + 1.21835i and the Second Root = -1.875 - 1.21835i
To solve complex quadratic problems with ease, use our quadratic formula calculator. It will make your calculation easier, faster, and more accurate.
A linear equation has only one solution or root. In the standard form of quadratic equation, if 'a' becomes 0, then the equation evaluates to bx + c = 0. Hence, it becomes a linear equation. The solution of a linear equation is given by:
The graph of a quadratic equation is basically a U-shaped curve called Parabola. The shape of the curve depends upon the sign of 'A' (the coefficient with x2).